Aircraft performance - making use of the airplane's capabilities and limitationsThis page discusses the factors that affect aircraft performance, which includes the airplane weight, atmospheric conditions, runway environment, and the fundamental physical laws governing the forces acting on an airplane. Importance of performance data The performance or operational information section of the Airplane Flight Manual/Pilot’s Operating Handbook (AFM/POH) contains the operating data for the airplane; that is, the data pertaining to takeoff, climb, range, endurance, descent, and landing. The use of this data in flying operations is mandatory for safe and efficient operation. Considerable knowledge and familiarity of the airplane can be gained through study of this material. It must be emphasized that the manufacturers’ information and data furnished in the AFM/POH is not standardized. Some provide the data in tabular form, while others use graphs. In addition, the performance data may be presented on the basis of standard atmospheric conditions, pressure altitude, or density altitude. The performance information in the AFM/POH has little or no value unless the user recognizes those variations and makes the necessary adjustments. To be able to make practical use of the airplane’s capabilities and limitations, it is essential to understand the significance of the operational data. The pilot must be cognizant of the basis for the performance data, as well as the meanings of the various terms used in expressing performance capabilities and limitations. Since the characteristics of the atmosphere have a predominant effect on performance, it is necessary to review some of the dominant factors—pressure and temperature. Structure of the atmosphere The atmosphere is an envelope of air that surrounds the earth and rests upon its surface. It is as much a part of the earth as the seas or the land. However, air differs from land and water inasmuch as it is a mixture of gases. It has mass, weight, and indefinite shape. Air, like any other fluid, is able to flow and change its shape when subjected to even minute pressures because of the lack of strong molecular cohesion. For example, gas will completely fill any container into which it is placed, expanding or contracting to adjust its shape to the limits of the container. The atmosphere is composed of 78 percent nitrogen, 21 percent oxygen, and 1 percent other gases, such as argon or helium. Most of the oxygen is contained below 35,000 feet altitude. Atmospheric pressure Though there are various kinds of pressure, pilots are mainly concerned with atmospheric pressure. It is one of the basic factors in weather changes, helps to lift the airplane, and actuates some of the important flight instruments in the airplane. These instruments are the altimeter, the airspeed indicator, the rate-of-climb indicator, and the manifold pressure gauge. Though air is very light, it has mass and is affected by the attraction of gravity. Therefore, like any other substance, it has weight, and because of its weight, it has force. Since it is a fluid substance, this force is exerted equally in all directions, and its effect on bodies within the air is called pressure. Under standard conditions at sea level, the average pressure exerted by the weight of the atmosphere is approximately 14.7 lb./in. The density of air has significant effects on the airplane’s performance. As air becomes less dense, it reduces:
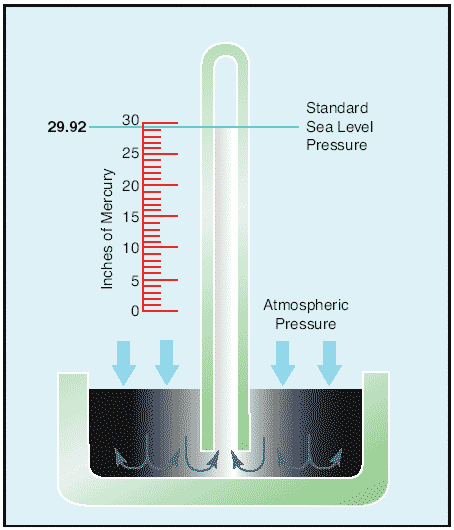
The pressure of the atmosphere varies with time and location. Due to the changing atmospheric pressure, a standard reference was developed. The standard atmosphere at sea level is a surface temperature of 59 °F or 15 °C and a surface pressure of 29.92 in. Hg or 1013.2 millibars.
Figure 1: Standard sea level pressure. A standard temperature lapse rate is one in which the temperature decreases at the rate of approximately 3.5 °F or 2 °C per thousand feet up to 36,000 feet. Above this point, the temperature is considered constant up to 80,000 feet. A standard pressure lapse rate is one in which pressure decreases at a rate of approximately 1 in. Hg per 1,000 feet of altitude gain to 10,000 feet.
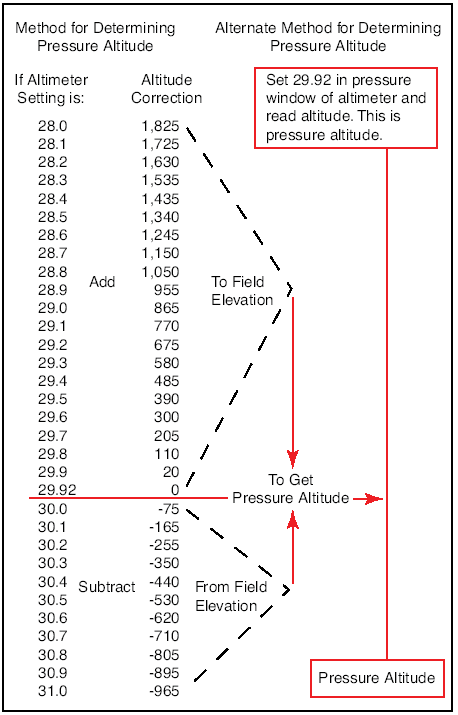
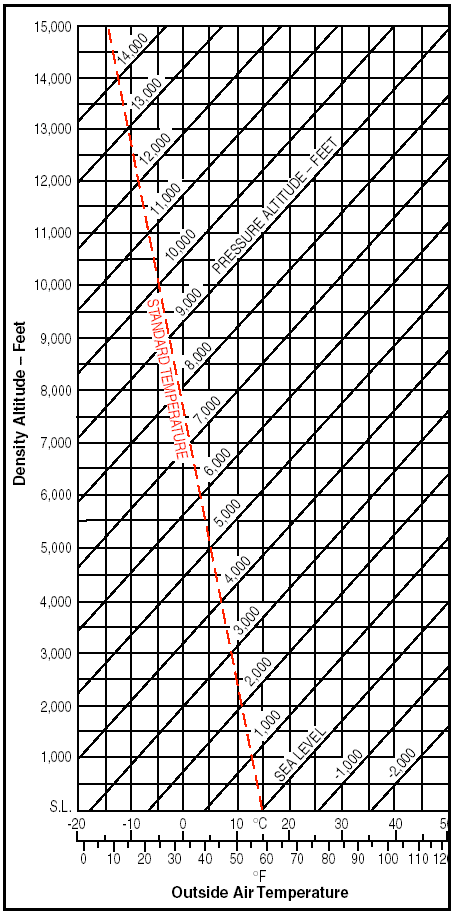
Figure 2: Properties of standard atmosphere. The International Civil Aviation Organization (ICAO) has established this as a worldwide standard, and it is often referred to as International Standard Atmosphere (ISA) or ICAO Standard Atmosphere. Any temperature or pressure that differs from the standard lapse rates is considered nonstandard temperature and pressure. Adjustments for nonstandard temperatures and pressures are provided on the manufacturer’s performance charts. Since all aircraft performance is compared and evaluated with respect to the standard atmosphere, all aircraft instruments are calibrated for the standard atmosphere. Thus, certain corrections must apply to the instrumentation, as well as the aircraft performance, if the actual operating conditions do not fit the standard atmosphere. In order to account properly for the nonstandard atmosphere, certain related terms must be defined. Pressure altitude Pressure altitude is the height above a standard datum plane. The airplane altimeter is essentially a sensitive barometer calibrated to indicate altitude in the standard atmosphere. If the altimeter is set for 29.92 in. Hg Standard Datum Plane (SDP), the altitude indicated is the pressure altitude—the altitude in the standard atmosphere corresponding to the sensed pressure. The SDP is a theoretical level where the weight of the atmosphere is 29.92 in. Hg as measured by a barometer. As atmospheric pressure changes, the SDP may be below, at, or above sea level. Pressure altitude is important as a basis for determining aircraft performance as well as for assigning flight levels to airplanes operating at above 18,000 feet. The pressure altitude can be determined by either of two methods: 1. by setting the barometric scale of the altimeter to 29.92 and reading the indicated altitude, or 2. by applying a correction factor to the indicated altitude according to the reported “altimeter setting.” Density altitude The more appropriate term for correlating aerodynamic performance in the nonstandard atmosphere is density altitude— the altitude in the standard atmosphere corresponding to a particular value of air density. Density altitude is pressure altitude corrected for nonstandard temperature. As the density of the air increases (lower density altitude), aircraft performance increases and conversely as air density decreases (higher density altitude), aircraft performance decreases. A decrease in air density means a high density altitude; and an increase in air density means a lower density altitude. Density altitude is used in calculating aircraft performance. Under standard atmospheric condition, air at each level in the atmosphere has a specific density, and under standard conditions, pressure altitude and density altitude identify the same level. Density altitude, then, is the vertical distance above sea level in the standard atmosphere at which a given density is to be found. The computation of density altitude must involve consideration of pressure (pressure altitude) and temperature. Since aircraft performance data at any level is based upon air density under standard day conditions, such performance data apply to air density levels that may not be identical with altimeter indications. Under conditions higher or lower than standard, these levels cannot be determined directly from the altimeter. Density altitude is determined by first finding pressure altitude, and then correcting this altitude for nonstandard temperature variations. Since density varies directly with pressure, and inversely with temperature, a given pressure altitude may exist for a wide range of temperature by allowing the density to vary. However, a known density occurs for any one temperature and pressure altitude. The density of the air, of course, has a pronounced effect on airplane and engine performance. Regardless of the actual altitude at which the airplane is operating, it will perform as though it were operating at an altitude equal to the existing density altitude. For example, when set at 29.92, the altimeter may indicate a pressure altitude of 5,000 feet. According to the AFM/POH, the ground run on takeoff may require a distance of 790 feet under standard temperature conditions. However, if the temperature is 20 °C above standard, the expansion of air raises the density level. Using temperature correction data from tables or graphs, or by deriving the density altitude with a computer, it may be found that the density level is above 7,000 feet, and the ground run may be closer to 1,000 feet. Air density is affected by changes in altitude, temperature, and humidity. High density altitude refers to thin air while low density altitude refers to dense air. The conditions that result in a high density altitude are high elevations, low atmospheric pressures, high temperatures, high humidity, or some combination of these factors. Lower elevations, high atmospheric pressure, low temperatures, and low humidity are more indicative of low density altitude. Using a flight computer, density altitude can be computed by inputting the pressure altitude and outside air temperature at flight level. Density altitude can also be determined by referring to the table and chart in figures 3 and 4.
Figure 3: Field elevation versus pressure altitude.
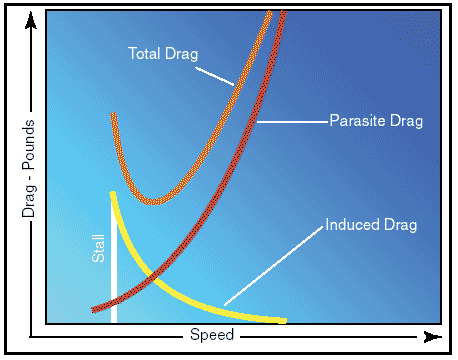
Figure 4: Density altitude chart. Effects of pressure on density Since air is a gas, it can be compressed or expanded. When air is compressed, a greater amount of air can occupy a given volume. Conversely, when pressure on a given volume of air is decreased, the air expands and occupies a greater space. That is, the original column of air at a lower pressure contains a smaller mass of air. In other words, the density is decreased. In fact, density is directly proportional to pressure. If the pressure is doubled, the density is doubled, and if the pressure is lowered, so is the density. This statement is true only at a constant temperature. Effect of temperature on density Increasing the temperature of a substance decreases its density. Conversely, decreasing the temperature increases the density. Thus, the density of air varies inversely with temperature. This statement is true only at a constant pressure. In the atmosphere, both temperature and pressure decrease with altitude, and have conflicting effects upon density. However, the fairly rapid drop in pressure as altitude is increased usually has the dominating effect. Hence, pilots can expect the density to decrease with altitude. Effect of humidity (moisture) on density The preceding paragraphs have assumed that the air was perfectly dry. In reality, it is never completely dry. The small amount of water vapor suspended in the atmosphere may be almost negligible under certain conditions, but in other conditions humidity may become an important factor in the performance of an airplane. Water vapor is lighter than air; consequently, moist air is lighter than dry air. Therefore, as the water content of the air increases, the air becomes less dense, increasing density altitude and decreasing performance. It is lightest or least dense when, in a given set of conditions, it contains the maximum amount of water vapor. Humidity, also called “relative humidity,” refers to the amount of water vapor contained in the atmosphere, and is expressed as a percentage of the maximum amount of water vapor the air can hold. This amount varies with the temperature; warm air can hold more water vapor, while colder air can hold less. Perfectly dry air that contains no water vapor has a relative humidity of 0 percent, while saturated air, that cannot hold any more water vapor, has a relative humidity of 100 percent. Humidity alone is usually not considered an important factor in calculating density altitude and airplane performance; however, it does contribute. The higher the temperature, the greater amount of water vapor that the air can hold. When comparing two separate air masses, the first warm and moist (both qualities tending to lighten the air) and the second cold and dry (both qualities making it heavier), the first necessarily must be less dense than the second. Pressure, temperature, and humidity have a great influence on aircraft performance because of their effect upon density. There are no rules-of-thumb or charts used to compute the effects of humidity on density altitude, so take this into consideration by expecting a decrease in overall performance in high humidity conditions. Performance “Performance” is a term used to describe the ability of an airplane to accomplish certain things that make it useful for certain purposes. For example, the ability of the airplane to land and take off in a very short distance is an important factor to the pilot who operates in and out of short, unimproved airfields. The ability to carry heavy loads, fly at high altitudes at fast speeds, or travel long distances is essential performance for operators of airline and executive type airplanes. The chief elements of performance are the takeoff and landing distance, rate of climb, ceiling, payload, range, speed, maneuverability, stability, and fuel economy. Some of these factors are often directly opposed: for example, high speed versus shortness of landing distance; long range versus great payload; and high rate of climb versus fuel economy. It is the preeminence of one or more of these factors which dictates differences between airplanes and which explains the high degree of specialization found in modern airplanes. The various items of aircraft performance result from the combination of airplane and powerplant characteristics. The aerodynamic characteristics of the airplane generally define the power and thrust requirements at various conditions of flight while powerplant characteristics generally define the power and thrust available at various conditions of flight. The matching of the aerodynamic configuration with the powerplant is accomplished by the manufacturer to provide maximum performance at the specific design condition; e.g. range, endurance, and climb. Straight-and-level flight All of the principal items of flight performance involve steady-state flight conditions and equilibrium of the airplane. For the airplane to remain in steady, level flight, equilibrium must be obtained by a lift equal to the airplane weight and a powerplant thrust equal to the airplane drag. Thus, the airplane drag defines the thrust required to maintain steady, level flight. All parts of the airplane that are exposed to the air contribute to the drag, though only the wings provide lift of any significance. For this reason, and certain others related to it, the total drag may be divided into two parts: the wing drag (induced) and the drag of everything but the wings (parasite). The total power required for flight then can be considered as the sum of induced and parasite effects; that is, the total drag of the airplane. Parasite drag is the sum of pressure and friction drag, which is due to the airplane’s basic configuration and, as defined, is independent of lift. Induced drag is the undesirable but unavoidable consequence of the development of lift. While the parasite drag predominates at high speed, induced drag predominates at low speed.
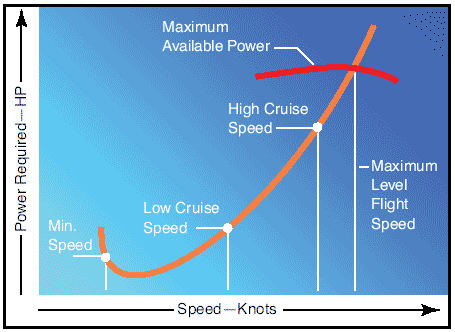
Figure 5: Drag versus speed. For example, if an airplane in a steady flight condition at 100 knots is then accelerated to 200 knots, the parasite drag becomes four times as great, but the power required to overcome that drag is eight times the original value. Conversely, when the airplane is operated in steady, level flight at twice as great a speed, the induced drag is one-fourth the original value, and the power required to overcome that drag is only one-half the original value. The wing or induced drag changes with speed in a very different way, because of the changes in the angle of attack. Near the stalling speed, the wing is inclined to the relative wind at nearly the stalling angle, and its drag is very strong. But at cruise flying speed, with the angle of attack nearly zero, induced drag is minimal. After attaining cruise speed, the angle of attack changes very little with any further increase in speed, and the drag of the wing increases in direct proportion to any further increase in speed. This does not consider the factor of compressibility drag that is involved at speeds beyond 260 knots. To sum up these changes, as the speed increases from stalling speed to VNE, the induced drag decreases and parasite drag increases. When the airplane is in steady, level flight, the condition of equilibrium must prevail. The unaccelerated condition of flight is achieved with the airplane trimmed for lift equal to weight and the powerplant set for a thrust to equal the airplane drag. The maximum level flight speed for the airplane will be obtained when the power or thrust required equals the maximum power or thrust available from the powerplant.
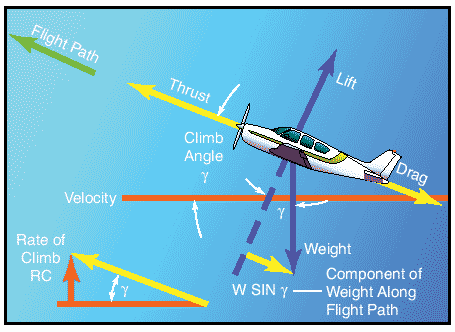
Figure 6: Power versus speed. The minimum level flight airspeed is not usually defined by thrust or power requirement since conditions of stall or stability and control problems generally predominate. Climb performance Climb depends upon the reserve power or thrust. Reserve power is the available power over and above that required to maintain horizontal flight at a given speed. Thus, if an airplane is equipped with an engine that produces 200 total available horsepower and the airplane requires only 130 horsepower at a certain level flight speed, the power available for climb is 70 horsepower. Although the terms “power” and “thrust” are sometimes used interchangeably, erroneously implying that they are synonymous, it is important to distinguish between the two when discussing climb performance. Work is the product of a force moving through a distance and is usually independent of time. Work is measured by several standards; the most common unit is called a “foot-pound.” If a 1-pound mass is raised 1 foot, a work unit of 1 foot-pound has been performed. The common unit of mechanical power is horsepower; one horsepower is work equivalent to lifting 33,000 pounds a vertical distance of 1 foot in 1 minute. The term “power” implies work rate or units of work per unit of time, and as such is a function of the speed at which the force is developed. “Thrust,” also a function of work, means the force that imparts a change in the velocity of a mass. This force is measured in pounds but has no element of time or rate. It can be said then, that during a steady climb, the rate of climb is a function of excess thrust. When the airplane is in steady, level flight or with a slight angle of climb, the vertical component of lift is very nearly the same as the actual total lift. Such climbing flight would exist with the lift very nearly equal to the weight. The net thrust of the powerplant may be inclined relative to the flightpath, but this effect will be neglected here for the sake of simplicity. Although the weight of the airplane acts vertically, a component of weight will act rearward along the flightpath.
Figure 7: Weight has rearward component. If it is assumed that the airplane is in a steady climb with essentially a small inclination of the flightpath, the summation of forces along the flightpath resolves to the following: Forces forward = Forces aft The basic relationship neglects some of the factors that may be of importance for airplanes of very high climb performance. (For example, a more detailed consideration would account for the inclination of thrust from the flightpath, lift not being equal to weight, and a subsequent change of induced drag.) However, this basic relationship will define the principal factors affecting climb performance. This relationship means that, for a given weight of the airplane, the angle of climb depends on the difference between thrust and drag, or the excess thrust.
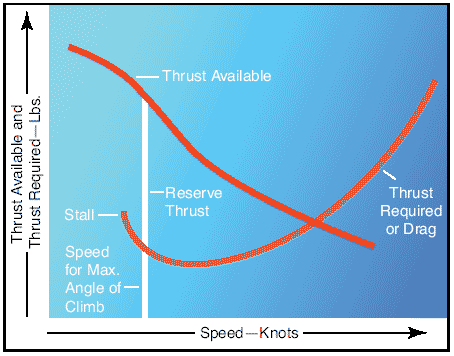
Figure 8: Thrust versus climb angle. Of course, when the excess thrust is zero, the inclination of the flightpath is zero, and the airplane will be in steady, level flight. When the thrust is greater than the drag, the excess thrust will allow a climb angle depending on the value of excess thrust. On the other hand, when the thrust is less than the drag, the deficiency of thrust will allow an angle of descent. The most immediate interest in the climb angle performance involves obstacle clearance. The most obvious purpose for which it might be used is to clear obstacles when climbing out of short or confined airports. The maximum angle of climb would occur where there exists the greatest difference between thrust available and thrust required; i.e., for the propeller-powered airplane, the maximum excess thrust and angle of climb will occur at some speed just above the stall speed. Thus, if it is necessary to clear an obstacle after takeoff, the propeller-powered airplane will attain maximum angle of climb at an airspeed close to—if not at—the takeoff speed. Of greater interest in climb performance are the factors that affect the rate of climb. The vertical velocity of an airplane depends on the flight speed and the inclination of the flightpath. In fact, the rate of climb is the vertical component of the flightpath velocity. For rate of climb, the maximum rate would occur where there exists the greatest difference between power available and power required.
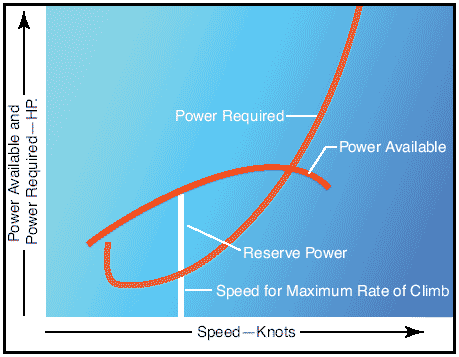
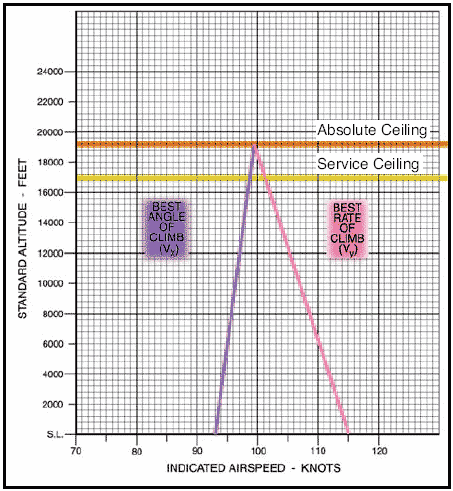
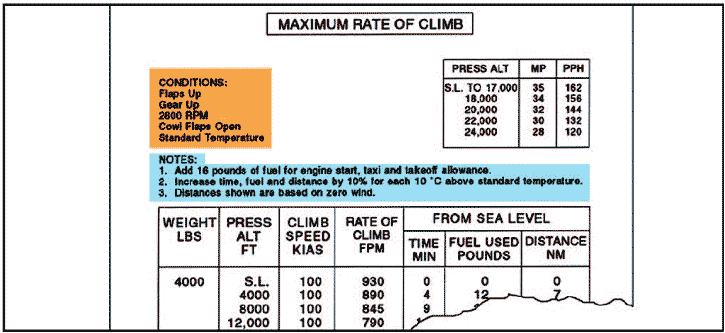
Figure 9: Power versus climb rate. The above relationship means that, for a given weight of the airplane, the rate of climb depends on the difference between the power available and the power required, or the excess power. Of course, when the excess power is zero, the rate of climb is zero and the airplane is in steady, level flight. When power available is greater than the power required, the excess power will allow a rate of climb specific to the magnitude of excess power. During a steady climb, the rate of climb will depend on excess power while the angle of climb is a function of excess thrust. The climb performance of an airplane is affected by certain variables. The conditions of the airplane’s maximum climb angle or maximum climb rate occur at specific speeds, and variations in speed will produce variations in climb performance. There is sufficient latitude in most airplanes that small variations in speed from the optimum do not produce large changes in climb performance, and certain operational considerations may require speeds slightly different from the optimum. Of course, climb performance would be most critical with high gross weight, at high altitude, in obstructed takeoff areas, or during malfunction of a powerplant. Then, optimum climb speeds are necessary. Weight has a very pronounced effect on aircraft performance. If weight is added to the airplane, it must fly at a higher angle of attack to maintain a given altitude and speed. This increases the induced drag of the wings, as well as the parasite drag of the airplane. Increased drag means that additional thrust is needed to overcome it, which in turn means that less reserve thrust is available for climbing. Airplane designers go to great effort to minimize the weight since it has such a marked effect on the factors pertaining to performance. A change in the airplane’s weight produces a twofold effect on climb performance. First, a change in weight will change the drag and the power required. This alters the reserve power available, which in turn, affects both the climb angle and the climb rate. Secondly, an increase in weight will reduce the maximum rate of climb, but the airplane must be operated at a higher climb speed to achieve the smaller peak climb rate. An increase in altitude also will increase the power required and decrease the power available. Therefore, the climb performance of an airplane diminishes with altitude. The speeds for maximum rate of climb, maximum angle of climb, and maximum and minimum level flight airspeeds vary with altitude. As altitude is increased, these various speeds finally converge at the absolute ceiling of the airplane. At the absolute ceiling, there is no excess of power and only one speed will allow steady, level flight. Consequently, the absolute ceiling of the airplane produces zero rate of climb. The service ceiling is the altitude at which the airplane is unable to climb at a rate greater than 100 feet per minute. Usually, these specific performance reference points are provided for the airplane at a specific design configuration.
Figure 10: Absolute and service ceiling. In discussing performance, it frequently is convenient to use the terms “power loading” and “wing loading.” Power loading is expressed in pounds per horsepower and is obtained by dividing the total weight of the airplane by the rated horsepower of the engine. It is a significant factor in the airplane’s takeoff and climb capabilities. Wing loading is expressed in pounds per square foot and is obtained by dividing the total weight of the airplane in pounds by the wing area (including ailerons) in square feet. It is the airplane’s wing loading that determines the landing speed. These factors are discussed in subsequent sections of this chapter. Range performance The ability of an airplane to convert fuel energy into flying distance is one of the most important items of aircraft performance. In flying operations, the problem of efficient range operation of an airplane appears in two general forms: 1. to extract the maximum flying distance from a given fuel load or, 2. to fly a specified distance with a minimum expenditure of fuel. A common denominator for each of these operating problems is the “specific range”; that is, nautical miles of flying distance per pound of fuel. Cruise flight operations for maximum range should be conducted so that the airplane obtains maximum specific range throughout the flight. The specific range can be defined by the following relationship:
or,
or,
If maximum specific range is desired, the flight condition must provide a maximum of speed per fuel flow. Range must be clearly distinguished from the item of endurance.
Figure 11: Airspeeds for maximum endurance vs. maximum range. The item of range involves consideration of flying distance, while endurance involves consideration of flying time. Thus, it is appropriate to define a separate term, “specific endurance.”
or,
or,
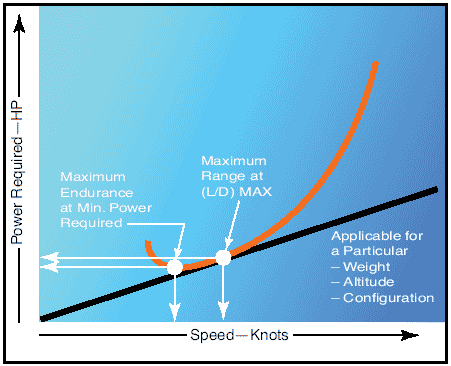
If maximum endurance is desired, the flight condition must provide a minimum of fuel flow. While the peak value of specific range would provide maximum range operation, long-range cruise operation is generally recommended at some slightly higher airspeed. Most long-range cruise operations are conducted at the flight condition that provides 99 percent of the absolute maximum specific range. The advantage of such operation is that 1 percent of range is traded for 3 to 5 percent higher cruise speed. Since the higher cruise speed has a great number of advantages, the small sacrifice of range is a fair bargain. The values of specific range versus speed are affected by three principal variables: 1. airplane gross weight, 2. altitude, and 3. the external aerodynamic configuration of the airplane. These are the source of range and endurance operating data included in the performance section of the AFM/POH. “Cruise control” of an airplane implies that the airplane is operated to maintain the recommended long-range cruise condition throughout the flight. Since fuel is consumed during cruise, the gross weight of the airplane will vary and optimum airspeed, altitude, and power setting can also vary. “Cruise control” means the control of the optimum airspeed, altitude, and power setting to maintain the 99 percent maximum specific range condition. At the beginning of cruise flight, the relatively high initial weight of the airplane will require specific values of airspeed, altitude, and power setting to produce the recommended cruise condition. As fuel is consumed and the airplane’s gross weight decreases, the optimum airspeed and power setting may decrease, or, the optimum altitude may increase. In addition, the optimum specific range will increase. Therefore, the pilot must provide the proper cruise control procedure to ensure that optimum conditions are maintained. Total range is dependent on both fuel available and specific range. When range and economy of operation are the principal goals, the pilot must ensure that the airplane will be operated at the recommended long- range cruise condition. By this procedure, the airplane will be capable of its maximum design-operating radius, or can achieve flight distances less than the maximum with a maximum of fuel reserve at the destination. The propeller-driven airplane combines the propeller with the reciprocating engine for propulsive power. In the case of the reciprocating engine, fuel flow is determined mainly by the shaft power put into the propeller rather than thrust. Thus, the fuel flow can be related directly to the power required to maintain the airplane in steady, level flight. This fact allows for the determination of range through analysis of power required versus speed. The maximum endurance condition would be obtained at the point of minimum power required since this would require the lowest fuel flow to keep the airplane in steady, level flight. Maximum range condition would occur where the proportion between speed and power required is greatest. [Figure 11] The maximum range condition is obtained at maximum lift/drag ratio (L/D max), and it is important to note that for a given airplane configuration, the maximum lift/drag ratio occurs at a particular angle of attack and lift coefficient, and is unaffected by weight or altitude. A variation in weight will alter the values of airspeed and power required to obtained the maximum lift/drag ratio.
Figure 12: Effect of weight on speed for maximum range. The variations of speed and power required must be monitored by the pilot as part of the cruise control procedure to maintain the maximum lift/drag ratio. When the airplane’s fuel weight is a small part of the gross weight and the airplane’s range is small, the cruise control procedure can be simplified to essentially maintaining a constant speed and power setting throughout the time of cruise flight. The long-range airplane has a fuel weight that is a considerable part of the gross weight, and cruise control procedures must employ scheduled airspeed and power changes to maintain optimum range conditions. The effect of altitude on the range of the propeller driven airplane may be understood by inspection of figure 13. A flight conducted at high altitude will have a greater true airspeed, and the power required will be proportionately greater than when conducted at sea level. The drag of the airplane at altitude is the same as the drag at sea level, but the higher true airspeed causes a proportionately greater power required. Note that the straight line that is tangent to the sea level power curve is also tangent to the altitude power curve.
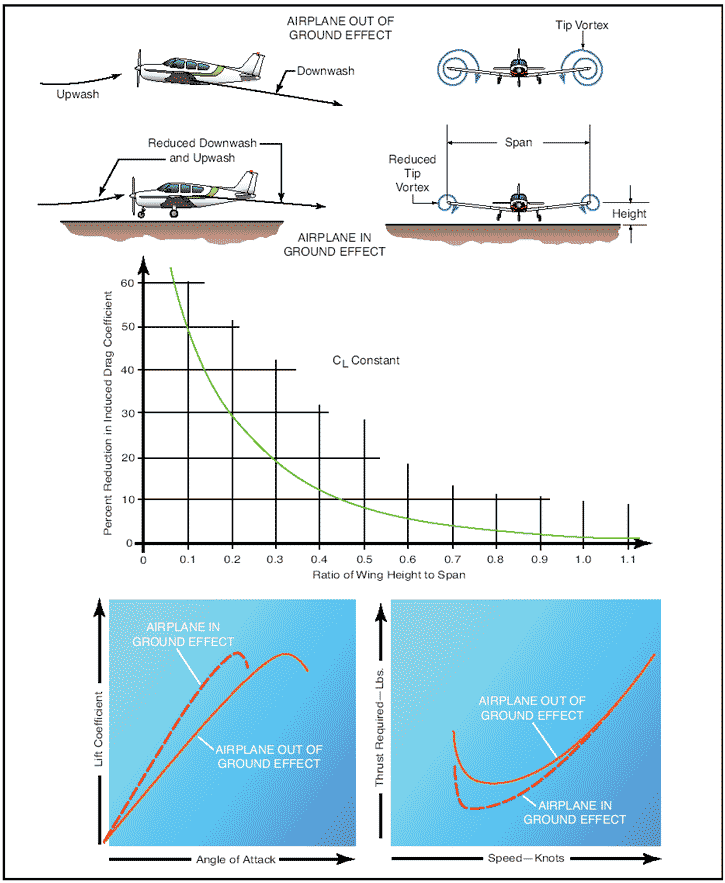
Figure 13: Effect of altitude on range. The effect of altitude on specific range also can be appreciated from the previous relationships. If a change in altitude causes identical changes in speed and power required, the proportion of speed to power required would be unchanged. The fact implies that the specific range of the propeller-driven airplane would be unaffected by altitude. Actually, this is true to the extent that specific fuel consumption and propeller efficiency are the principal factors that could cause a variation of specific range with altitude. If compressibility effects are negligible, any variation of specific range with altitude is strictly a function of engine/propeller performance. The airplane equipped with the reciprocating engine will experience very little, if any, variation of specific range up to its absolute altitude. There is negligible variation of brake specific fuel consumption for values of brake horsepower below the maximum cruise power rating of the engine that is the lean range of engine operation. Thus, an increase in altitude will produce a decrease in specific range only when the increased power requirement exceeds the maximum cruise power rating of the engine. One advantage of supercharging is that the cruise power may be maintained at high altitude, and the airplane may achieve the range at high altitude with the corresponding increase in true airspeed. The principal differences in the high altitude cruise and low altitude cruise are the true airspeeds and climb fuel requirements. Ground effect Ground effect is due to the interference of the surface with the flow pattern about the airplane in flight. Ground effect can be detected and measured up to an altitude equal to one wing span above the surface. However, ground effect is most significant when the airplane (especially the low-wing airplane) is maintaining a constant attitude at low airspeed and low altitude (for example, during landing flare before touchdown, and during takeoff when the airplane lifts off and accelerates to climb speed). When the wing is under the influence of ground effect, there is a reduction in upwash, downwash, and tip vortices. As a result of the reduced tip vortices, induced drag is reduced. When the wing is at a height equal to one-fourth the span, the reduction in induced drag is about 25 percent, and when the wing is at a height equal to one-tenth the span, the reduction in induced drag is about 50 percent. At high speeds where parasite drag predominates, induced drag is a small part of the total drag. Consequently, the effects of ground effect are of greater concern during takeoff and landing.
Figure 14: Ground effect. Assuming that the airplane descends into ground effect maintaining a constant angle of attack and a constant airspeed, the following effects will take place: Because of the reduction in drag, a smaller wing angle of attack will be required to produce the same lift coefficient or, if a constant wing angle of attack is maintained, the wing will experience an increase in lift coefficient. As a result of the reduction in drag, the thrust required at low speeds will be reduced. The reduction in downwash at the horizontal tail will reduce the effectiveness of the elevator. It may cause a pitch-down tendency, thus requiring greater up elevator to trim the airplane. In the majority of cases, ground effect will cause an increase in pressure at the static source and produce a lower indication of airspeed and altitude. During the landing flare when the airplane is brought into ground effect at a constant angle of attack, the airplane will experience an increase in lift coefficient. Thus, a “floating” sensation may be experienced. Because of the reduced drag in ground effect, any excess speed at the point of landing flare may result in a considerable “float” distance. If a power approach is being made, the power setting should be reduced as the airplane descends into ground effect to avoid overshooting the desired touchdown point. During takeoff, the airplane leaving ground effect encounters the reverse of entering ground effect. For example, an airplane leaving ground effect will:
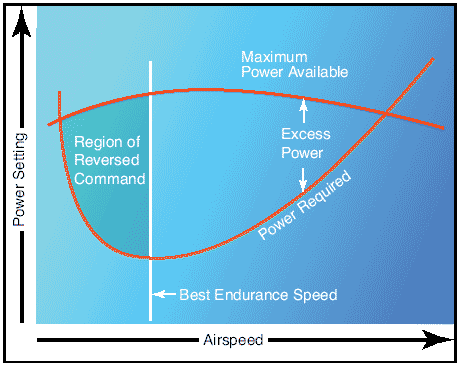
Due to the reduced drag in ground effect, the airplane may seem able to take off below the recommended airspeed. However, as the airplane rises out of ground effect with an insufficient airspeed, initial climb performance may prove to be marginal because of the increased drag. Under extreme conditions such as high density altitude, high temperature, and maximum gross weight, the airplane may be able to become airborne at an insufficient airspeed, but unable to fly out of ground effect. Consequently, the airplane may not be able to clear an obstruction, or may settle back on the runway. Under marginal conditions, it is important the airplane takes off at the recommended speed that will provide adequate initial climb performance. If the runway is long enough, or no obstacles exist, ground effect can be used to an advantage by using the reduced drag to improve initial acceleration. Ground effect is important to normal flight operations in the performance of soft and rough field takeoffs and landings. The procedure for takeoff from these surfaces is to transfer as much weight as possible to the wings during the ground run, and to lift off with the aid of ground effect before true flying speed is attained. It is then necessary to reduce the angle of attack gradually until normal airspeed is attained before attempting to climb away from the ground effect. Region of reversed command The aerodynamic properties of the airplane generally determine the power requirements at various conditions of flight, while the powerplant capabilities generally determine the power available at various conditions of flight. When the airplane is in steady, level flight, the condition of equilibrium must prevail. An unaccelerated condition of flight is achieved when lift equals weight, and the powerplant is set for a thrust equal to the airplane drag. The power required to achieve equilibrium in constant-altitude flight at various airspeeds is depicted on a power required curve. The power required curve illustrates the fact that at low airspeeds near the stall or minimum controllable airspeed, the power setting required for steady, level flight is quite high. Flight in the region of normal command means that while holding a constant altitude, a higher airspeed requires a higher power setting and a lower airspeed requires a lower power setting. The majority of all airplane flying (climb, cruise, and maneuvers) is conducted in the region of normal command. Flight in the region of reversed command means that a higher airspeed requires a lower power setting and a lower airspeed requires a higher power setting to hold altitude. It does not imply that a decrease in power will produce lower airspeed. The region of reversed command is encountered in the low speed phases of flight. Flight speeds below the speed for maximum endurance (lowest point on the power curve) require higher power settings with a decrease in airspeed. Since the need to increase the required power setting with decreased speed is contrary to the normal command of flight, the regime of flight speeds between the speed for minimum required power setting and the stall speed (or minimum control speed) is termed the region of reversed command. In the region of reversed command, a decrease in airspeed must be accompanied by an increased power setting in order to maintain steady flight.
Figure 15: Power required curve. Figure 15 shows the “maximum power available” as a curved line. Lower power settings, such as cruise power, would also appear in a similar curve. The lowest point on the power required curve represents the speed at which the lowest brake horsepower will sustain level flight. This is termed the best endurance airspeed. An airplane performing a low airspeed, high pitch attitude power approach for a short-field landing is an example of operating in the region of reversed command. If an unacceptably high sink rate should develop, it may be possible for the pilot to reduce or stop the descent by applying power. But without further use of power, the airplane would probably stall or be incapable of flaring for the landing. Merely lowering the nose of the airplane to regain flying speed in this situation, without the use of power, would result in a rapid sink rate and corresponding loss of altitude. If during a soft-field takeoff and climb, for example, the pilot attempts to climb out of ground effect without first attaining normal climb pitch attitude and airspeed, the airplane may inadvertently enter the region of reversed command at a dangerously low altitude. Even with full power, the airplane may be incapable of climbing or even maintaining altitude. The pilot’s only recourse in this situation is to lower the pitch attitude in order to increase airspeed, which will inevitably result in a loss of altitude. Airplane pilots must give particular attention to precise control of airspeed when operating in the low flight speeds of the region of reversed command. Runway surface and gradient Runway conditions affect takeoff and landing performance. Typically, performance chart information assumes paved, level, smooth, and dry runway surfaces. Since no two runways are alike, the runway surface differs from one runway to another, as does the runway gradient or slope.
Figure 16: Charts assume paved, level, dry runway conditions. Runway surfaces vary widely from one airport to another. The runway surface encountered may be concrete, asphalt, gravel, dirt, or grass. The runway surface for a specific airport is noted in the Airport/Facility Directory. Any surface that is not hard and smooth will increase the ground roll during takeoff. This is due to the inability of the tires to smoothly roll along the runway. Tires can sink into soft, grassy, or muddy runways. Potholes or other ruts in the pavement can be the cause of poor tire movement along the runway. Obstructions such as mud, snow, or standing water reduce the airplane’s acceleration down the runway. Although muddy and wet surface conditions can reduce friction between the runway and the tires, they can also act as obstructions and reduce the landing distance.
Figure 17: Aircraft performance depends greatly on the runway surface. Braking effectiveness is another consideration when dealing with various runway types. The condition of the surface affects the braking ability of the airplane. The amount of power that is applied to the brakes without skidding the tires is referred to as braking effectiveness. Ensure that runways are adequate in length for takeoff acceleration and landing deceleration when less than ideal surface conditions are being reported. The gradient or slope of the runway is the amount of change in runway height over the length of the runway. The gradient is expressed as a percentage such as a 3 percent gradient. This means that for every 100 feet of runway length, the runway height changes by 3 feet. A positive gradient indicates that the runway height increases, and a negative gradient indicates that the runway decreases in height. An upsloping runway impedes acceleration and results in a longer ground run during takeoff. However, landing on an upsloping runway typically reduces the landing roll. A downsloping runway aids in acceleration on takeoff resulting in shorter takeoff distances. The opposite is true when landing, as landing on a downsloping runway increases landing distances. Runway slope information is contained in the Airport/Facility Directory.
Figure 18: A/FDs provide information regarding runway slope. Water on the runway and dynamic hydroplaning Water on the runways reduces the friction between the tires and the ground, and can reduce braking effectiveness. The ability to brake can be completely lost when the tires are hydroplaning because a layer of water separates the tires from the runway surface. This is also true of braking effectiveness when runways are covered in ice. When the runway is wet, the pilot may be confronted with dynamic hydroplaning. Dynamic hydroplaning is a condition in which the airplane tires ride on a thin sheet of water rather than on the runway’s surface. Because hydroplaning wheels are not touching the runway, braking and directional control are almost nil. To help minimize dynamic hydroplaning, some runways are grooved to help drain off water; but most runways are not.
Figure 19: Dynamic hydroplane formula and example for a tire pressure of 36 pounds. Tire pressure is a factor in dynamic hydroplaning. By the simple formula in figure 19, the pilot can calculate the minimum speed, in knots, at which hydroplaning will begin. In plain language, the minimum hydroplaning speed is determined by multiplying the square root of the main gear tire pressure in pounds per square inch (p.s.i.), by nine. For example, if the main gear tire pressure is at 36 pounds per square inch, the airplane would begin hydroplaning at 54 knots. Landing at higher than recommended touchdown speeds will expose the airplane to a greater potential for hydroplaning. And once hydroplaning starts, it can continue well below the minimum, initial hydroplaning speed. On wet runways, directional control can be maximized by landing into the wind. Abrupt control inputs should be avoided. When the runway is wet, anticipate braking problems well before landing and be prepared for hydroplaning. Opt for a suitable runway most aligned with the wind. Mechanical braking may be ineffective, so aerodynamic braking should be used to its fullest advantage. Takeoff and landing performance The majority of pilot-caused airplane accidents occur during the takeoff and landing phase of flight. Because of this fact, the pilot must be familiar with all the variables that influence the takeoff and landing performance of an airplane and must strive for exacting, professional procedures of operation during these phases of flight. Takeoff and landing performance is a condition of accelerated and decelerated motion. For instance, during takeoff, the airplane starts at zero speed and accelerates to the takeoff speed to become airborne. During landing, the airplane touches down at the landing speed and decelerates to zero speed. The important factors of takeoff or landing performance are as follows:
Takeoff performance The minimum takeoff distance is of primary interest in the operation of any airplane because it defines the runway requirements. The minimum takeoff distance is obtained by taking off at some minimum safe speed that allows sufficient margin above stall and provides satisfactory control and initial rate of climb. Generally, the lift-off speed is some fixed percentage of the stall speed or minimum control speed for the airplane in the takeoff configuration. As such, the lift-off will be accomplished at some particular value of lift coefficient and angle of attack. Depending on the airplane characteristics, the lift-off speed will be anywhere from 1.05 to 1.25 times the stall speed or minimum control speed. To obtain minimum takeoff distance at the specific lift-off speed, the forces that act on the airplane must provide the maximum acceleration during the takeoff roll. The various forces acting on the airplane may or may not be under the control of the pilot, and various procedures may be necessary in certain airplanes to maintain takeoff acceleration at the highest value. The powerplant thrust is the principal force to provide the acceleration and, for minimum takeoff distance, the output thrust should be at a maximum. Lift and drag are produced as soon as the airplane has speed, and the values of lift and drag depend on the angle of attack and dynamic pressure. In addition to the important factors of proper procedures, many other variables affect the takeoff performance of an airplane. Any item that alters the takeoff speed or acceleration rate during the takeoff roll will affect the takeoff distance. For example, the effect of gross weight on takeoff distance is significant and proper consideration of this item must be made in predicting the airplane’s takeoff distance. Increased gross weight can be considered to produce a threefold effect on takeoff performance: 1. higher lift-off speed, 2. greater mass to accelerate, and 3. increased retarding force (drag and ground friction). If the gross weight increases, a greater speed is necessary to produce the greater lift necessary to get the airplane airborne at the takeoff lift coefficient. As an example of the effect of a change in gross weight, a 21 percent increase in takeoff weight will require a 10 percent increase in lift-off speed to support the greater weight. A change in gross weight will change the net accelerating force and change the mass that is being accelerated. If the airplane has a relatively high thrust-to-weight ratio, the change in the net accelerating force is slight and the principal effect on acceleration is due to the change in mass. The takeoff distance will vary at least as the square of the gross weight. For example, a 10 percent increase in takeoff gross weight would cause:
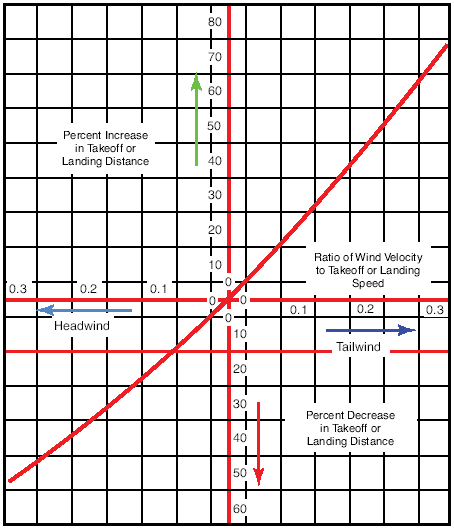
For the airplane with a high thrust-to-weight ratio, the increase in takeoff distance might be approximately 21 to 22 percent, but for the airplane with a relatively low thrust-to-weight ratio, the increase in takeoff distance would be approximately 25 to 30 percent. Such a powerful effect requires proper consideration of gross weight in predicting takeoff distance. The effect of wind on takeoff distance is large, and proper consideration also must be provided when predicting takeoff distance. The effect of a headwind is to allow the airplane to reach the lift-off speed at a lower groundspeed while the effect of a tailwind is to require the airplane to achieve a greater groundspeed to attain the lift-off speed. A headwind that is 10 percent of the takeoff airspeed will reduce the takeoff distance approximately 19 percent. However, a tailwind that is 10 percent of the takeoff airspeed will increase the takeoff distance approximately 21 percent. In the case where the headwind speed is 50 percent of the takeoff speed, the takeoff distance would be approximately 25 percent of the zero wind takeoff distance (75 percent reduction). The effect of wind on landing distance is identical to the effect on takeoff distance. Figure 20 illustrates the general effect of wind by the percent change in takeoff or landing distance as a function of the ratio of wind velocity to takeoff or landing speed.
Figure 20: Effect of wind on takeoff and landing. The effect of proper takeoff speed is especially important when runway lengths and takeoff distances are critical. The takeoff speeds specified in the AFM/POH are generally the minimum safe speeds at which the airplane can become airborne. Any attempt to take off below the recommended speed could mean that the airplane may stall, be difficult to control, or have a very low initial rate of climb. In some cases, an excessive angle of attack may not allow the airplane to climb out of ground effect. On the other hand, an excessive airspeed at takeoff may improve the initial rate of climb and “feel” of the airplane, but will produce an undesirable increase in takeoff distance. Assuming that the acceleration is essentially unaffected, the takeoff distance varies as the square of the takeoff velocity. Thus, 10 percent excess airspeed would increase the takeoff distance 21 percent. In most critical takeoff conditions, such an increase in takeoff distance would be prohibitive, and the pilot must adhere to the recommended takeoff speeds. The effect of pressure altitude and ambient temperature is to define primarily the density altitude and its effect on takeoff performance. While subsequent corrections are appropriate for the effect of temperature on certain items of powerplant performance, density altitude defines specific effects on takeoff performance. An increase in density altitude can produce a twofold effect on takeoff performance: 1. greater takeoff speed and 2. decreased thrust and reduced net accelerating force. If an airplane of given weight and configuration is operated at greater heights above standard sea level, the airplane will still require the same dynamic pressure to become airborne at the takeoff lift coefficient. Thus, the airplane at altitude will take off at the same indicated airspeed as at sea level, but because of the reduced air density, the true airspeed will be greater. The effect of density altitude on powerplant thrust depends much on the type of powerplant. An increase in altitude above standard sea level will bring an immediate decrease in power output for the unsupercharged reciprocating engine. However, an increase in altitude above standard sea level will not cause a decrease in power output for the supercharged reciprocating engine until the altitude exceeds the critical operating altitude. For those powerplants that experience a decay in thrust with an increase in altitude, the effect on the net accelerating force and acceleration rate can be approximated by assuming a direct variation with density. Actually, this assumed variation would closely approximate the effect on airplanes with high thrust-to-weight ratios. Proper accounting of pressure altitude (field elevation is a poor substitute) and temperature is mandatory for accurate prediction of takeoff roll distance. The most critical conditions of takeoff performance are the result of some combination of high gross weight, altitude, temperature, and unfavorable wind. In all cases, the pilot must make an accurate prediction of takeoff distance from the performance data of the AFM/POH, regardless of the runway available, and strive for a polished, professional takeoff procedure. In the prediction of takeoff distance from the AFM/POH data, the following primary considerations must be given:
Landing performance In many cases, the landing distance of an airplane will define the runway requirements for flying operations. The minimum landing distance is obtained by landing at some minimum safe speed, which allows sufficient margin above stall and provides satisfactory control and capability for a go-around. Generally, the landing speed is some fixed percentage of the stall speed or minimum control speed for the airplane in the landing configuration. As such, the landing will be accomplished at some particular value of lift coefficient and angle of attack. The exact values will depend on the airplane characteristics but, once defined, the values are independent of weight, altitude, and wind. To obtain minimum landing distance at the specified landing speed, the forces that act on the airplane must provide maximum deceleration during the landing roll. The forces acting on the airplane during the landing roll may require various procedures to maintain landing deceleration at the peak value. A distinction should be made between the procedures for minimum landing distance and an ordinary landing roll with considerable excess runway available. Minimum landing distance will be obtained by creating a continuous peak deceleration of the airplane; that is, extensive use of the brakes for maximum deceleration. On the other hand, an ordinary landing roll with considerable excess runway may allow extensive use of aerodynamic drag to minimize wear and tear on the tires and brakes. If aerodynamic drag is sufficient to cause deceleration of the airplane, it can be used in deference to the brakes in the early stages of the landing roll; i.e., brakes and tires suffer from continuous hard use, but airplane aerodynamic drag is free and does not wear out with use. The use of aerodynamic drag is applicable only for deceleration to 60 or 70 percent of the touchdown speed. At speeds less than 60 to 70 percent of the touchdown speed, aerodynamic drag is so slight as to be of little use, and braking must be utilized to produce continued deceleration of the airplane. Since the objective during the landing roll is to decelerate, the powerplant thrust should be the smallest possible positive value (or largest possible negative value in the case of thrust reversers). In addition to the important factors of proper procedures, many other variables affect the landing performance. Any item that alters the landing speed or deceleration rate during the landing roll will affect the landing distance. The effect of gross weight on landing distance is one of the principal items determining the landing distance. One effect of an increased gross weight is that a greater speed will be required to support the airplane at the landing angle of attack and lift coefficient. For an example of the effect of a change in gross weight, a 21 percent increase in landing weight will require a 10 percent increase in landing speed to support the greater weight. When minimum landing distances are considered, braking friction forces predominate during the landing roll and, for the majority of airplane configurations, braking friction is the main source of deceleration. The minimum landing distance will vary in direct proportion to the gross weight. For example, a 10 percent increase in gross weight at landing would cause a:
A contingency of this is the relationship between weight and braking friction force. The effect of wind on landing distance is large and deserves proper consideration when predicting landing distance. Since the airplane will land at a particular airspeed independent of the wind, the principal effect of wind on landing distance is due to the change in the groundspeed at which the airplane touches down. The effect of wind on deceleration during the landing is identical to the effect on acceleration during the takeoff. A headwind that is 10 percent of the landing airspeed will reduce the landing distance approximately 19 percent, but a tailwind that is 10 percent of the landing speed will increase the landing distance approximately 21 percent. Figure 20 illustrates this general effect. The effect of pressure altitude and ambient temperature is to define density altitude and its effect on landing performance. An increase in density altitude will increase the landing speed but will not alter the net retarding force. Thus, the airplane at altitude will land at the same indicated airspeed as at sea level but, because of the reduced density, the true airspeed (TAS) will be greater. Since the airplane lands at altitude with the same weight and dynamic pressure, the drag and braking friction throughout the landing roll have the same values as at sea level. As long as the condition is within the capability of the brakes, the net retarding force is unchanged, and the deceleration is the same as with the landing at sea level. Since an increase in altitude does not alter deceleration, the effect of density altitude on landing distance would actually be due to the greater TAS. The minimum landing distance at 5,000 feet would be 16 percent greater than the minimum landing distance at sea level. The approximate increase in landing distance with altitude is approximately 3 1/2 percent for each 1,000 feet of altitude. Proper accounting of density altitude is necessary to accurately predict landing distance. The effect of proper landing speed is important when runway lengths and landing distances are critical. The landing speeds specified in the AFM/POH are generally the minimum safe speeds at which the airplane can be landed. Any attempt to land at below the specified speed may mean that the airplane may stall, be difficult to control, or develop high rates of descent. On the other hand, an excessive speed at landing may improve the controllability slightly (especially in crosswinds), but will cause an undesirable increase in landing distance. A 10 percent excess landing speed would cause at least a 21 percent increase in landing distance. The excess speed places a greater working load on the brakes because of the additional kinetic energy to be dissipated. Also, the additional speed causes increased drag and lift in the normal ground attitude, and the increased lift will reduce the normal force on the braking surfaces. The deceleration during this range of speed immediately after touchdown may suffer, and it will be more likely that a tire can be blown out from braking at this point. The most critical conditions of landing performance are the result of some combination of high gross weight, high density altitude, and unfavorable wind. These conditions produce the greatest landing distance and provide critical levels of energy dissipation required of the brakes. In all cases, it is necessary to make an accurate prediction of minimum landing distance to compare with the available runway. A polished, professional landing procedure is necessary because the landing phase of flight accounts for more pilot-caused airplane accidents than any other single phase of flight. In the prediction of minimum landing distance from the AFM/POH data, the following considerations must be given:
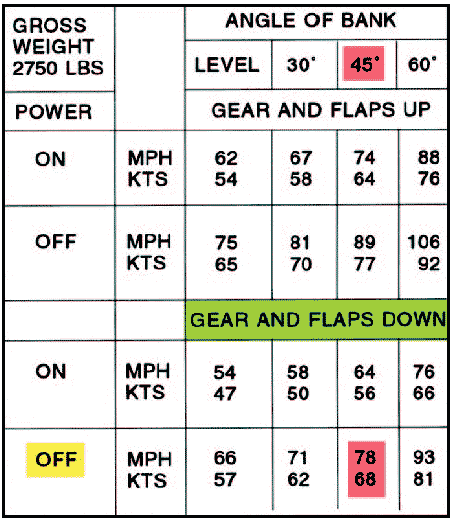
Performance speeds True Airspeed (TAS) the speed of the airplane in relation to the air mass in which it is flying. Indicated Airspeed (IAS) the speed of the airplane as observed on the airspeed indicator. It is the airspeed without correction for indicator, position (or installation), or compressibility errors. Calibrated Airspeed (CAS) the airspeed indicator reading corrected for position (or installation), and instrument errors. (CAS is equal to TAS at sea level in standard atmosphere.) The color-coding for various design speeds marked on airspeed indicators may be IAS or CAS. Equivalent Airspeed (EAS) the airspeed indicator reading corrected for position (or installation), or instrument error, and for adiabatic compressible flow for the particular altitude. (EAS is equal to CAS at sea level in standard atmosphere.) VS0 the calibrated power-off stalling speed or the minimum steady flight speed at which the airplane is controllable in the landing configuration. VS1 the calibrated power-off stalling speed or the minimum steady flight speed at which the airplane is controllable in a specified configuration. VY the calibrated airspeed at which the airplane will obtain the maximum increase in altitude per unit of time. This best rate-of-climb speed normally decreases slightly with altitude. VX the calibrated airspeed at which the airplane will obtain the highest altitude in a given horizontal distance. This best angle-of-climb speed normally increases slightly with altitude. VLE the maximum calibrated airspeed at which the airplane can be safely flown with the landing gear extended. This is a problem involving stability and controllability. VLO the maximum calibrated airspeed at which the landing gear can be safely extended or retracted. This is a problem involving the air loads imposed on the operating mechanism during extension or retraction of the gear. VFE the highest calibrated airspeed permissible with the wing flaps in a prescribed extended position. This is because of the air loads imposed on the structure of the flaps. VA the calibrated design maneuvering airspeed. This is the maximum speed at which the limit load can be imposed (either by gusts or full deflection of the control surfaces) without causing structural damage. VNO the maximum calibrated airspeed for normal operation or the maximum structural cruising speed. This is the speed at which exceeding the limit load factor may cause permanent deformation of the airplane structure. VNE the calibrated airspeed which should NEVER be exceeded. If flight is attempted above this speed, structural damage or structural failure may result. Performance charts Performance charts allow a pilot to predict the takeoff, climb, cruise, and landing performance of the airplane. These charts, provided by the manufacturer, are included in the AFM/POH. The information the manufacturer provides on these charts has been gathered from test flights conducted in a new airplane, under normal operating conditions while using average piloting skills, and with the airplane and engine in good working order. Engineers record the flight data and create performance charts based on the behavior of the airplane during the test flights. By using these performance charts, a pilot can determine the runway length needed to take off and land, the amount of fuel that will be used during flight, and the length of time it will take to arrive at the destination. It is important to remember that the data from the charts will not be accurate if the airplane is not in good working order or when operating under adverse conditions. So take into consideration that it is necessary to compensate the performance numbers if the airplane is not in good working order or piloting skills are below average. Each airplane performs differently and therefore, has different performance numbers. Compute the performance of the airplane prior to every flight, as every flight is different. Every chart is based on certain conditions and contains notes on how to adapt the information for flight conditions. It is important to read every chart and understand how to use it. Read the accompanying instructions provided by the manufacturer. For an explanation on how to use the charts, refer to the example provided by the manufacturer for that specific chart.
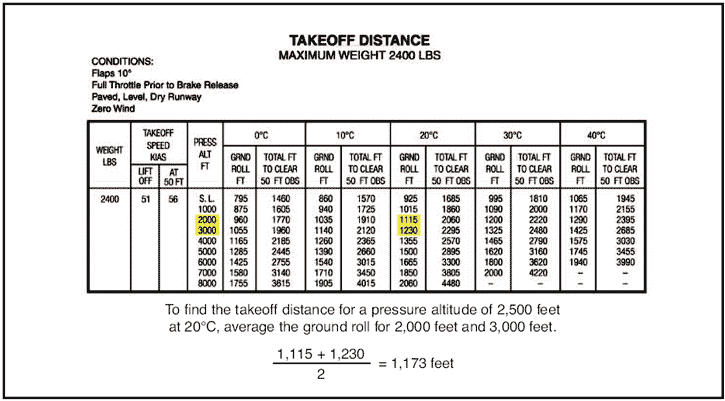
Figure 21: Carefully read all conditions and notes for every chart. The information manufacturers furnish is not standardized. Information may be contained in a table format, and other information may be contained in a graph format. Sometimes combined graphs incorporate two or more graphs into one chart to compensate for multiple conditions of flight. Combined graphs allow the pilot to predict aircraft performance for variations in density altitude, weight, and winds all on one chart. Because of the vast amount of information that can be extracted from this type of chart, it is important to be very accurate in reading the chart. A small error in the beginning can lead to a large error at the end. The remainder of this section covers performance information for airplanes in general and discusses what information the charts contain and how to extract information from the charts by direct reading and interpolation methods. Every chart contains a wealth of information that should be used when flight planning. Examples of the table, graph, and combined graph formats for all aspects of flight will be discussed. Interpolation Not all of the information on the charts is easily extracted. Some charts require interpolation to find the information for specific flight conditions. Interpolating information means that by taking the known information, a pilot can compute intermediate information. However, pilots sometimes round off values from charts to a more conservative figure. Using values that reflect slightly more adverse conditions provides a reasonable estimate of performance information and gives a slight margin of safety. The following illustration is an example of interpolating information from a takeoff distance chart.
Figure 22: Interpolating charts. Density altitude charts Use a density altitude chart to figure the density altitude at the departing airport. Using figure 23, determine the density altitude based on the given information. Sample Problem 1
Figure 23: Density altitude chart.
First, compute the pressure altitude conversion. Find 30.10 under the altimeter heading. Read across to the second column. It reads “-165.” Therefore, it is necessary to subtract 165 from the airport elevation giving a pressure altitude of 5,718 feet. Next, locate the outside air temperature on the scale along the bottom of the graph. From 70°, draw a line up to the 5,718 feet pressure altitude line, which is about two-thirds of the way up between the 5,000 and 6,000-foot lines. Draw a line straight across to the far left side of the graph and read the approximate density altitude. The approximate density altitude in thousands of feet is 7,700 feet. Takeoff charts Takeoff charts are typically provided in several forms. They allow a pilot to compute the takeoff distance of the airplane with no flaps or with a specific flap configuration. A pilot can also compute distances for a no flap takeoff over a 50-foot obstacle scenario as well as with flaps over a 50-foot obstacle. The takeoff distance chart provides for various airplane weights, altitudes, temperatures, winds, and obstacle heights. Sample Problem 2
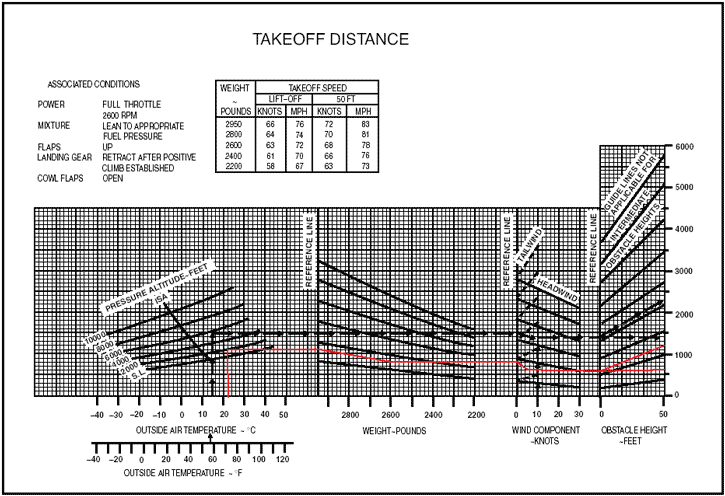
Figure 24: Combined takeoff distance graph. Refer to figure 24. This chart is an example of a combined takeoff distance graph. It takes into consideration pressure altitude, temperature, weight, wind, and obstacles all on one chart. First, find the correct temperature on the bottom left-hand side of the graph. Follow the line from 22°C straight up until it intersects the 2,000-foot altitude line. From that point, draw a line straight across to the first dark reference line. Continue to draw the line from the reference point in a diagonal direction following the surrounding lines until it intersects the corresponding weight line. From the intersection of 2,600 pounds, draw a line straight across until it reaches the second reference line. Once again, follow the lines in a diagonal manner until it reaches the 6-knot headwind mark. Follow straight across to the third reference line and from here, draw a line in two directions. First, draw a line straight across to figure the ground roll distance. Next, follow the diagonal lines again until it reaches the corresponding obstacle height. In this case, it is a 50-foot obstacle. Therefore, draw the diagonal line to the far edge of the chart. This results in a 600-foot ground roll distance and a total distance of 1,200 feet over a 50-foot obstacle. To find the corresponding takeoff speeds at lift-off and over the 50-foot obstacle, refer to the table on the top of the chart. In this case, the lift-off speed at 2,600 pounds would be 63 knots and over the 50-foot obstacle would be 68 knots. Sample Problem 3
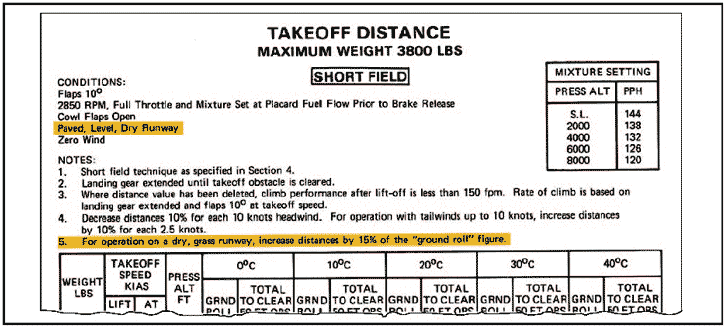
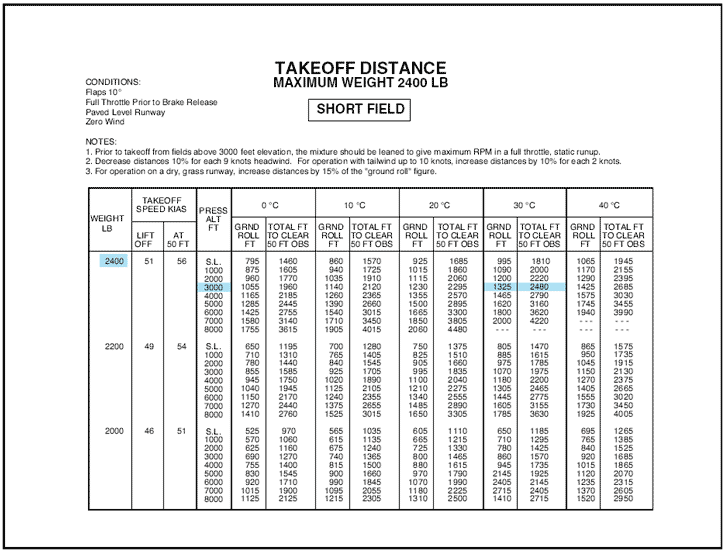
Figure 25: Takeoff distance table, short-field. Refer to figure 25. This chart is an example of a takeoff distance table for short-field takeoffs. For this table, first find the takeoff weight. Once at 2,400 pounds, begin reading from left to right across the table. The takeoff speed is in the second column and, in the third column under pressure altitude, find the pressure altitude of 3,000 feet. Carefully follow that line to the right until it is under the correct temperature column of 30°C. The ground roll total reads 1,325 feet and the total required to clear a 50-foot obstacle is 2,480 feet. At this point, there are 18 knots of headwind. Reading in the notes section under point number two, it says to decrease the distances by 10 percent for each 9 knots of headwind. With 18 knots of headwind, it is necessary to decrease the distance by 20 percent. Multiply 1,325 feet by 20 percent or by .20 (1325 x .20 = 265), then subtract that amount from the total distance (1325 – 265 = 1060). Repeat this process for the total distance over a 50-foot obstacle. The ground roll distance is 1,060 feet and the total distance over a 50-foot obstacle is 1,984 feet. Climb and cruise charts Climb and cruise chart information is based on actual flight tests conducted in an airplane of the same type. This information is extremely useful when planning a cross-country to predict the performance and fuel consumption of the airplane. Manufacturers produce several different charts for climb and cruise performance. These charts will include everything from fuel, time, and distance to climb, to best power setting during cruise, to cruise range performance. The first chart to check for climb performance is a fuel, time, and distance-to-climb chart. This chart will give the fuel amount used during the climb, the time it will take to accomplish the climb, and the ground distance that will be covered during the climb. To use this chart, obtain the information for the departing airport and for the cruise altitude. Using figure 26, calculate the fuel, time, and distance to climb based on the information provided.
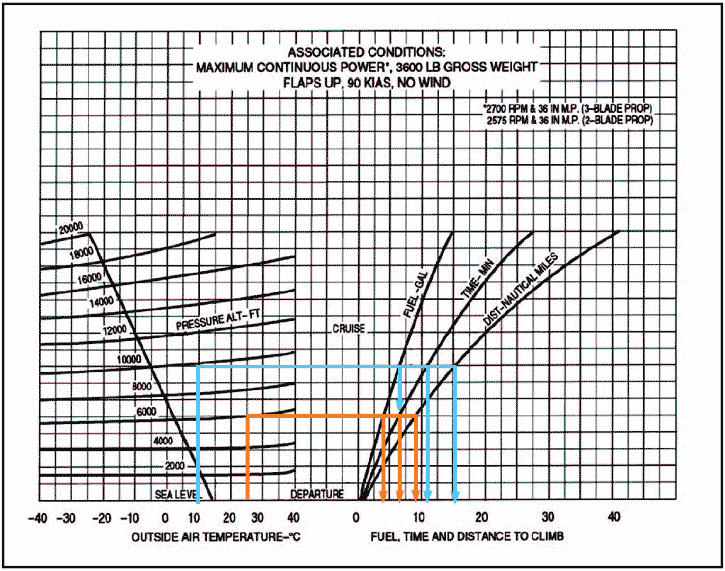
Figure 26: Fuel, time, and distance-to-climb chart. Sample Problem 4
First, find the information for the departing airport. Find the OAT for the departing airport along the bottom, left-hand side of the graph. Follow the line from 25°C straight up until it intersects the line corresponding to the pressure altitude of 6,000 feet. Continue this line straight across until it intersects all three lines for fuel, time, and distance. Draw a line straight down from the intersection of altitude and fuel, altitude and time, and a third line at altitude and distance. It should read 3.5 gallons of fuel, 6.5 minutes of time, and 9 nautical miles. Next, repeat the steps to find the information for the cruise altitude. It should read 6.5 gallons of fuel, 11.5 minutes of time, and 15 nautical miles. Take each set of numbers for fuel, time, and distance and subtract them from one another (6.5 - 3.5 = 3 gallons of fuel). It will take 3 gallons of fuel and 5 minutes of time to climb to 10,000 feet. During that climb, the distance covered is 6 nautical miles. Remember, according to the notes at the top of the chart, these numbers do not take into account wind, and it is assumed maximum continuous power is being used. The next example is a fuel, time, and distance-to-climb table. For this table, use the same basic criteria as for the previous chart. However, it is necessary to figure the information in a different manner. Refer to figure 27 to work the following sample problem.
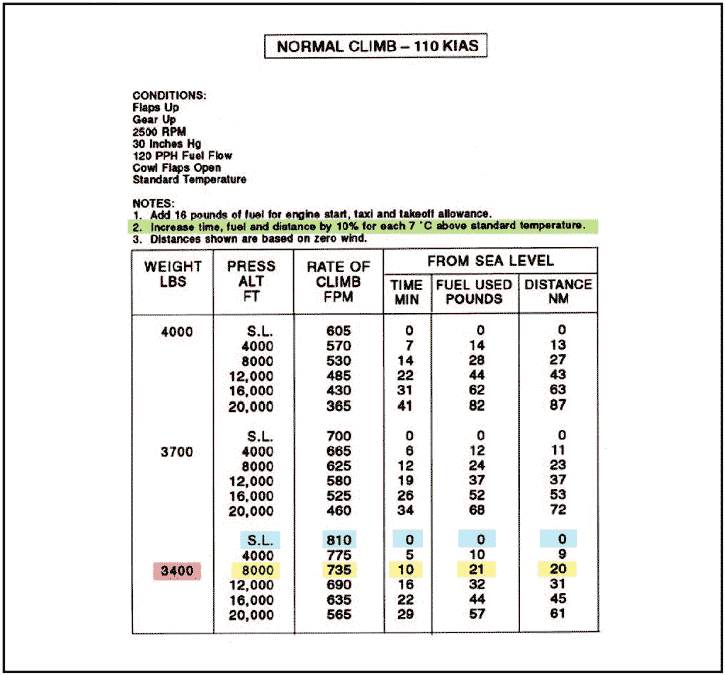
Figure 27: Fuel, time, and distance-to-climb table. Sample Problem 5
To begin, find the given weight of 3,400 in the first column of the chart. Move across to the pressure altitude column to find the sea level altitude numbers. At sea level, the numbers read zero. Next, read the line that corresponds with the cruising altitude of 8,000 feet. Normally, a pilot would subtract these two sets of number from one another, but given the fact that the numbers read zero at sea level, it is known that the time to climb from sea level to 8,000 feet is 10 minutes. It is also known that 21 pounds of fuel will be used and 20 nautical miles will be covered during the climb. However, the temperature is 22°C, which is 7º above the standard temperature of 15°C. The notes section of this chart indicate that our findings must be increased by 10 percent for each 7º above standard. Multiply the findings by 10 percent or .10 (10 x .10 = 1, 1 + 10 = 11 minutes). After accounting for the additional 10 percent, the findings should read 11 minutes, 23.1 pounds of fuel, and 22 nautical miles. Notice that the fuel is reported in pounds of fuel, not gallons. Aviation fuel weighs 6 pounds per gallon, so 23.1 pounds of fuel is equal to 3.85 gallons of fuel (23.1 / 6 = 3.85). The next example is a cruise and range performance chart. This type of table is designed to give true airspeed, fuel consumption, endurance in hours, and range in miles at specific cruise configurations. Use figure 28 to determine the cruise and range performance under the given conditions.
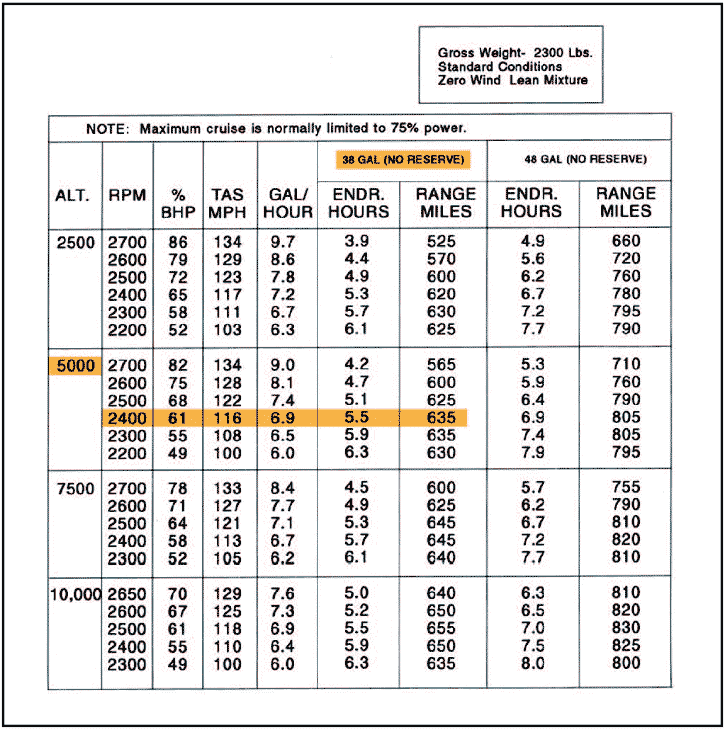
Figure 28: Cruise and range performance. Sample Problem 6
Find 5,000 feet pressure altitude in the first column on the left-hand side of the table. Next, find the correct r.p.m. of 2,400 in the second column. Follow that line straight across and read the TAS of 116 m.p.h., and a fuel burn rate of 6.9 gallons per hour. As per the example, the airplane is equipped with a fuel carrying capacity of 38 gallons. Under this column, read that the endurance in hours is 5.5 hours and the range in miles is 635 miles. Cruise power setting tables are useful when planning cross-country flights. The table gives the correct cruise power settings as well as the fuel flow and airspeed performance numbers at that altitude and airspeed. Sample Problem 7
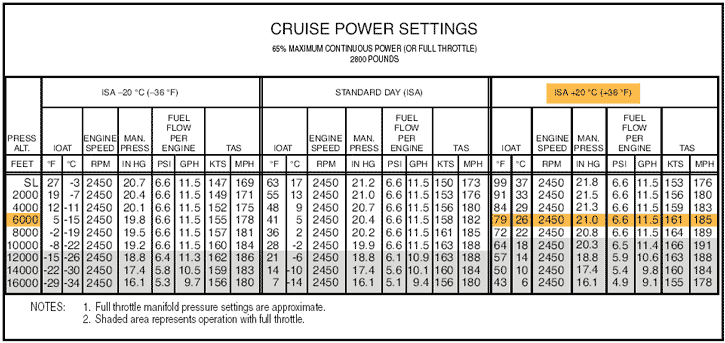
Figure 29: Cruise power setting table. Refer to figure 29 for this sample problem. First, locate the pressure altitude of 6,000 feet on the far left side of the table. Follow that line across to the far right side of the table under the 20°C (or 36°F) column. At 6,000 feet, the r.p.m. setting of 2,450 will maintain 65 percent continuous power at 21.0 inches of manifold pressure with a fuel flow rate of 11.5 gallons per hour and airspeed of 161 knots.
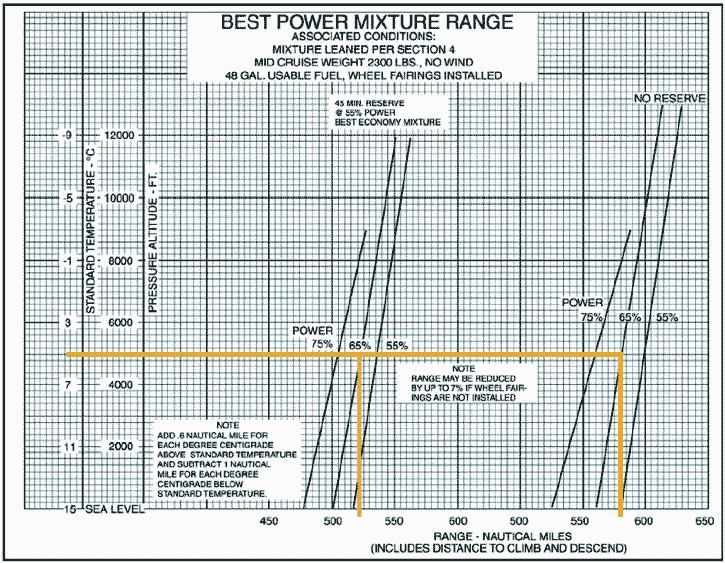
Figure 30: Best power mixture range graph. Another type of cruise chart is a best power mixture range graph. This graph gives the best range based on power setting and altitude. Using figure 30, find the range at 65 percent power with and without a reserve based on the provided conditions. Sample Problem 8
First, move up the left side of the graph to 5,000 feet and standard temperature. Follow the line straight across the graph until it intersects the 65 percent line under both the reserve and no reserve categories. Draw a line straight down from both intersections to the bottom of the graph. At 65 percent power with a reserve, the range is approximately 522 miles. At 65 percent power with no reserve, the range should be 581 miles. The last cruise chart referenced is a cruise performance graph. This graph is designed to tell the true airspeed (TAS) performance of the airplane depending on the altitude, temperature, and power setting. Using figure 31, find the TAS performance based on the given information.
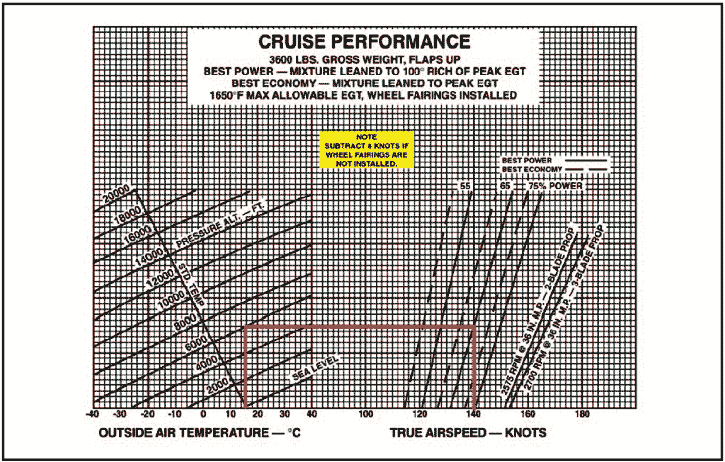
Figure 31: Cruise performance graph. Sample Problem 9
Begin by finding the correct OAT on the bottom, left-hand side of the graph. Move up that line until it intersects the pressure altitude of 6,000 feet. Draw a line straight across to the 65 percent, best power line. This is the solid line, not the dashed line, which represents best economy. Draw a line straight down from this intersection to the bottom of the graph. The true airspeed at 65 percent best power is 140 knots. However, it is necessary to subtract 8 knots from the speed since there are no wheel fairings. This note is listed under the title and conditions. The true airspeed will be 132 knots. Crosswind and headwind component chart Every airplane is tested according to FAA regulations prior to certification. The airplane is tested by a pilot with average piloting skills in 90 ° crosswinds with a velocity up to 0.2 VSO or two-tenths of the airplane’s stalling speed with power off, gear down, and flaps down. This means that if the stalling speed of the airplane is 45 knots, it must be capable of being landed in a 9 knot, 90 ° crosswind. The maximum demonstrated crosswind component is published in the AFM/POH. The crosswind and headwind component chart allows for figuring the headwind and crosswind component for any given wind direction and velocity. Sample Problem 10
Figure 32: Crosswind component chart. Refer to figure 32 to solve this problem. First, determine how many degrees difference there is between the runway and the wind direction. It is known that runway 17 means a direction of 170° and from that, subtract the wind direction of 140°. This gives a 30° angular difference. This is the wind angle. Next, locate the 30° mark and draw a line from there until it intersects the correct wind velocity of 25 knots. From there, draw a line straight down and a line straight across. The headwind component is 22 knots and the crosswind component is 13 knots. This information is important when taking off and landing so that, first of all, the appropriate runway can be picked if more than one exists at a particular airport, but also so that the airplane is not pushed beyond its tested limits. Landing charts Landing performance is affected by variables similar to those affecting takeoff performance. It is necessary to compensate for differences in density altitude, weight of the airplane, and headwinds. Like takeoff performance charts, landing distance information is available as normal landing information as well as landing distance over a 50-foot obstacle. As usual, read the associated conditions and notes in order to ascertain the basis of the chart information. Remember, when calculating landing distance that the landing weight will not be the same as the takeoff weight. The weight must be recalculated to compensate for the fuel that was used during the flight. Sample Problem 11
Figure 33: Landing distance table. Refer to figure 33. This example makes use of a landing distance table. Notice that the altitude of 1,250 feet is not on this table. It is therefore necessary to use interpolation skills to find the correct landing distance. The pressure altitude of 1,250 is halfway between sea level and 2,500 feet. First, find the column for sea level and the column for 2,500 feet. Take the total distance of 1,075 for sea level and the total distance of 1,135 for 2,500 and add them together. Divide the total by two to obtain the distance for 1,250 feet. The distance is 1,105 feet total landing distance to clear a 50-foot obstacle. Repeat this process to obtain the ground roll distance for the pressure altitude. The ground roll should be 457.5 feet. Sample Problem 12
Figure 34: Landing distance graph. Using the given conditions and figure 34, determine the landing distance for the airplane. This graph is an example of a combined landing distance graph and allows compensation for temperature, weight, headwinds, tailwinds, and varying obstacle height. Begin by finding the correct OAT on the Fahrenheit scale on the left-hand side of the chart. Move up in a straight line to the correct pressure altitude of 4,000 feet. From this intersection, move straight across to the first dark reference line. Follow the lines in the same diagonal fashion until the correct landing weight is reached. At 2,400 pounds, continue in a straight line across to the second dark reference line. Once again, draw a line in a diagonal manner to the correct wind component and then straight across to the third dark reference line. From this point, draw a line in two separate directions: one straight across to figure the ground roll and one in a diagonal manner to the correct obstacle height. This should be 900 feet for the total ground roll and 1,300 feet for the total distance over a 50-foot obstacle. Stall speed performance charts Stall speed performance charts are designed to give an understanding of the speed at which the airplane will stall in a given configuration. This type of chart will typically take into account the angle of bank, the position of the gear and flaps, and the throttle position. Use figure 35 and the accompanying conditions to find the speed at which the airplane will stall.
Figure 35: Stall speed table. Sample Problem 13
First, locate the correct flap and gear configuration. The bottom half of the chart should be used since the gear and flaps are down. Next, choose the row corresponding to a power-off situation. Now find the correct angle of bank column, which is 45°. The stall speed in miles per hour (m.p.h.) is 78 m.p.h., and the stall speed in knots would be 68 knots. Performance charts provide valuable information to the pilot. Take advantage of these charts. A pilot can predict the performance of the airplane under most flying conditions, and this enables a better plan for every flight. The Code of Federal Regulations (CFR) requires that a pilot be familiar with all information available prior to any flight. Pilots should use the information to their advantage as it can only contribute to safety in flight. Transport category aircraft performance Transport category airplanes are certificated under Title 14 of the Code of Federal Regulations (14 CFR) part 25. The airworthiness certification standards of part 25 require proven levels of performance and guaranteed safety margins for these airplanes, regardless of the specific operating regulations under which they are employed. Major differences in transport category versus non-transport category performance requirements
All of the performance charts for the transport category airplanes require that takeoff and climb performance be computed with the full effects of temperature considered.
The transport category airplane’s climb performance is expressed as a percent gradient of climb rather than a figure calculated in feet per minute of climb. This percent gradient of climb is a much more practical expression of performance since it is the airplane’s angle of climb that is critical in an obstacle clearance situation.
Lift-off technique in transport category airplanes allows the reaching of V2 (takeoff safety speed) after the airplane is airborne. This is possible because of the excellent acceleration and reliability characteristics of the engines on these airplanes and also because of the larger surplus of power.
All airplanes certificated by the FAA in the transport category, whatever the size, must be operated in accordance with the same performance criteria. This applies to both commercial and non-commercial operations. Performance requirements The performance requirements that the transport category airplane must meet are as follows: Takeoff
Landing
Takeoff planning The following are the speeds that affect the transport category airplane’s takeoff performance. The flight crew must be thoroughly familiar with each of these speeds and how they are used in takeoff planning. Speed Definition VS Stalling speed or the minimum steady flight speed at which the airplane is controllable. VMCG Minimum control speed on the ground, with one engine inoperative, (critical engine on two-engine airplanes) takeoff power on other engine(s), using aerodynamic controls only for directional control. (Must be less than V1). VMCA Minimum control speed in the air, with one engine inoperative, (critical engine on two-engine airplanes) operating engine(s) at take off power, maximum of 5° bank into the good engine(s). V1 Critical engine failure speed or decision speed. Engine failure below this speed shall result in an aborted takeoff; above this speed the take off run should be continued. VR Speed at which the rotation of the airplane is initiated to takeoff attitude. This speed cannot be less than V1 or less than 1.05 times VMC. With an engine failure, it must also allow for the acceleration to V2 at the 35-foot height at the end of the runway. VLO Lift-off speed. The speed at which the airplane first becomes airborne. V2 The takeoff safety speed which must be attained at the 35-foot height at the end of the required runway distance. This is essentially the best one-engine inoperative angle of climb speed for the airplane and should be held until clearing obstacles after takeoff, or until at least 400 feet above the ground. VFS Final segment climb speed, which is based upon one-engine inoperative climb, clean configuration, and maximum continuous power setting. All of the above V speeds should be considered during every takeoff. The V1, VR, V2 and VFS speeds should be visibly posted in the cockpit for reference during the takeoff. Takeoff speeds vary with airplane weight. Before takeoff speeds can be computed, the pilot must first determine the maximum allowable takeoff weight. The three items that can limit takeoff weight are runway requirements, takeoff climb requirements, and obstacle clearance requirements. Runway requirements The runway requirements for takeoff will be affected by the following:
The runway required for takeoff must be based upon the possible loss of an engine at the most critical point, which is at V1 (decision speed). By regulation, the airplane’s takeoff weight has to accommodate the longest of three distances: 1. Accelerate-Go Distance The distance required to accelerate to V1 with all engines at takeoff power, experience an engine failure at V1 and continue the takeoff on the remaining engine(s). The runway required includes the distance required to climb to 35 feet by which time V2 speed must be attained. 2. Accelerate-Stop Distance The distance required to accelerate to V1 with all engines at takeoff power, experience an engine failure at V1, and abort the takeoff and bring the airplane to a stop using braking action only (use of thrust reversing is not considered). 3. Takeoff Distance The distance required to complete an all-engines operative takeoff to the 35-foot height. It must be at least 15 percent less than the distance required for a one-engine inoperative engine takeoff. This distance is not normally a limiting factor as it is usually less than the one-engine inoperative takeoff distance. These three required takeoff runway considerations are shown in figure 36.
Figure 36: Minimum required takeoff runway lengths. Balanced field length In most cases, the pilot will be working with a performance chart for takeoff runway required, which will give “balanced field length” information. This means that the distance shown for the takeoff will include both the accelerate-go and accelerate-stop distances. One effective means of presenting the normal takeoff data is shown in the tabulated chart in figure 37.
Figure 37: Normal takeoff runway required. The chart in figure 37 shows the runway distance required under normal conditions and is useful as a quick reference chart for the standard takeoff. The V speeds for the various weights and conditions are also shown. For other than normal takeoff conditions, such as with engine anti-ice, anti-skid brakes inoperative, or extremes in temperature or runway slope, the pilot should consult the appropriate takeoff performance charts in the performance section of the Airplane Flight Manual. There are other occasions of very high weight and temperature where the runway requirement may be dictated by the maximum brake kinetic energy limits that affect the airplane’s ability to stop. Under these conditions, the accelerate-stop distance may be greater than the accelerate-go. The procedure to bring performance back to a balanced field takeoff condition is to limit the V1 speed so that it does not exceed the maximum brake kinetic energy speed (sometimes called VBE). This procedure also results in a reduction in allowable takeoff weight. Climb requirements After the airplane has reached the 35-foot height with one engine inoperative, there is a requirement that it be able to climb at a specified climb gradient. This is known as the takeoff flightpath requirement. The airplane’s performance must be considered based upon a one-engine inoperative climb up to 1,500 feet above the ground. The takeoff flightpath profile with required gradients of climb for the various segments and configurations is shown in figure 38.
Figure 38: One-engine inoperative takeoff flightpath. Note: Climb gradient can best be described as being a certain gain of vertical height for a given distance covered horizontally. For instance, a 2.4 percent gradient means that 24 feet of altitude would be gained for each 1,000 feet of distance covered horizontally across the ground. The following brief explanation of the one-engine inoperative climb profile may be helpful in understanding the chart in figure 38. First segment This segment is included in the takeoff runway required charts and is measured from the point at which the airplane becomes airborne until it reaches the 35-foot height at the end of the runway distance required. Speed initially is VLO and must be V2 at the 35-foot height. Second segment This is the most critical segment of the profile. The second segment is the climb from the 35-foot height to 400 feet above the ground. The climb is done at full takeoff power on the operating engine(s), at V2 speed, and with the flaps in the takeoff configuration. The required climb gradient in this segment is 2.4 percent for two-engine airplanes, 2.7 percent for three-engine airplanes, and 3.0 percent for four-engine airplanes. Third or acceleration segment During this segment, the airplane is considered to be maintaining the 400 feet above the ground and accelerating from the V2 speed to the VFS speed before the climb profile is continued. The flaps are raised at the beginning of the acceleration segment and power is maintained at the takeoff setting as long as possible (5 minutes maximum). Fourth or final segment This segment is from the 400 to 1,500-foot AGL altitude with power set at maximum continuous. The required climb in this segment is a gradient of 1.2 percent for two-engine airplanes, 1.55 for three-engine airplanes, and 1.7 percent for four-engine airplanes. Second segment climb limitations The second segment climb requirements, from 35 to 400 feet, are the most restrictive (or hardest to meet) of the climb segments. The pilot must determine that the second segment climb is met for each takeoff. In order to achieve this performance at the higher density altitude conditions, it may be necessary to limit the takeoff weight of the airplane. It must be realized that, regardless of the actual available length of the takeoff runway, takeoff weight must be adjusted so that the second segment climb requirements can be met. The airplane may well be capable of lifting off with one engine inoperative, but it must then be able to climb and clear obstacles. Although second segment climb may not present much of a problem at the lower altitudes, at the higher altitude airports and higher temperatures the second segment climb chart should be consulted to determine the effects on maximum takeoff weights before figuring takeoff runway distance required. Air carrier obstacle clearance requirements Regulations require that large transport category turbine powered airplanes certificated after September 30, 1958, be taken off at a weight that allows a net takeoff flightpath (one engine inoperative) that clears all obstacles either by a height of at least 35 feet vertically, or by at least 200 feet horizontally within the airport boundaries and by at least 300 feet horizontally after passing the boundaries. The takeoff flightpath is considered to begin 35 feet above the takeoff surface at the end of the takeoff distance, and extends to a point in the takeoff at which the airplane is 1,500 feet above the takeoff surface, or at which the transition from the takeoff to the enroute configuration is completed. The net takeoff flightpath is the actual takeoff flightpath reduced at each point by 0.8 percent for two-engine airplanes, 0.9 percent for three-engine airplanes, and 1.0 percent for four-engine airplanes. Air carrier pilots therefore are responsible not only for determining that there is enough runway available for an engine inoperative takeoff (balanced field length), and the ability to meet required climb gradients; but they must also assure that the airplane will be able to safely clear any obstacles that may be in the takeoff flightpath. The net takeoff flightpath and obstacle clearance required are shown in figure 39.
Figure 39: Takeoff obstacle clearance requirements. The usual method of computing net takeoff flightpath performance is to add up the total ground distances required for each of the climb segments and/or use obstacle clearance performance charts in the AFM. Although this obstacle clearance requirement is seldom a limitation at the normally used airports, it is quite often an important consideration under critical conditions such as high takeoff weight and/or high-density altitude. Consider that at a 2.4 percent climb gradient (2.4 feet up for every 100 feet forward) a 1,500-foot altitude gain would take a horizontal distance of 10.4 nautical miles to achieve. Summary of takeoff requirements In order to establish the allowable takeoff weight for a transport category airplane, at any airfield, the following must be considered:
Once the above details are known and applied to the appropriate performance charts, it is possible to determine the maximum allowable takeoff weight. This weight would be the lower of the maximum weights as allowed by:
In practice, restrictions to takeoff weight at low altitude airports are usually due to runway length limitations; engine inoperative climb limitations are most common at the higher altitude airports. All limitations to weight must be observed. Since the combined weight of fuel and payload in the airplane may amount to nearly half the maximum takeoff weight, it is usually possible to reduce fuel weight to meet takeoff limitations. If this is done, however, flight planning must be recalculated in light of reduced fuel and range. Landing performance As in the takeoff planning, certain speeds must be considered during landing. These speeds are shown below. Level condition Speed Definition VSO Stalling speed or the minimum steady flight speed in the landing configuration. VREF 1.3 times the stalling speed in the landing configuration. This is the required speed at the 50-foot height above the threshold end of the runway. Approach Climb The approach climb speed is the speed which would give the best climb performance in the approach configuration, with one engine inoperative, and with maximum takeoff power on the operating engine(s). The required gradient of climb in this configuration is 2.1 percent for two-engine airplanes, 2.4 percent for three-engine airplanes, and 2.7 percent for four-engine airplanes. Landing Climb This speed would give the best performance in the full landing configuration with maximum takeoff power on all engines. The gradient of climb required in this configuration is 3.2 percent. Planning the landing As in the takeoff, the landing speeds shown above should be precomputed and visible to both pilots prior to the landing. The VREF speed, or threshold speed, is used as a reference speed throughout the traffic pattern or instrument approach as in the following example:
Landing requirements The maximum landing weight of an airplane can be restricted by either the approach climb requirements or by the landing runway available. Approach climb requirements The approach climb is usually more limiting (or more difficult to meet) than the landing climb, primarily because it is based upon the ability to execute a missed approach with one engine inoperative. The required climb gradient can be affected by pressure altitude and temperature and, as in the second segment climb in the takeoff, airplane weight must be limited as needed in order to comply with this climb requirement. Landing runway required The runway distance needed for landing can be affected by the following:
In computing the landing distance required, some manufacturers do not include all of the above items in their charts, since the regulations state that only pressure altitude, wind, and airplane weight must be considered. Charts are provided for anti-skid on and anti-skid off conditions, but the use of reverse thrust is not used in computing required landing distances. The landing distance, as required by the regulations, is that distance needed to land and come to a complete stop from a point 50 feet above the threshold end of the runway. It includes the air distance required to travel from the 50-foot height to touchdown (which can consume 1,000 feet of runway distance), plus the stopping distance, with no margin left over. This is all that is required for 14 CFR part 91 operators (non-air carrier), and all that is shown on some landing distance required charts. For air carriers and other commercial operators subjected to 14 CFR part 121, a different set of rules applies which states that the required landing distance from the 50-foot height cannot exceed 60 percent of the actual runway length available. In all cases, the minimum airspeed allowed at the 50-foot height must be no less than 1.3 times the airplane’s stalling speed in the landing configuration. This speed is commonly called the airplane’s VREF speed and will vary with landing weight. Figure 40 is a diagram of these landing runway requirements.
Figure 40: Landing runway requirements. Summary of landing requirements In order to establish the allowable landing weight for a transport category airplane, the following details must be considered:
With these details, it is possible to establish the maximum allowable landing weight, which will be the lower of the weights as dictated by:
In practice, the approach climb limitations (ability to climb in approach configuration with one engine inoperative) are seldom encountered because the landing weights upon arrival at the destination airport are usually light. However, as in the second segment climb requirement for takeoff, this approach climb gradient must be met and landing weights must be restricted if necessary. The most likely conditions that would make the approach climb critical would be the landings at high weights and high-pressure altitudes and temperatures, which might be encountered if a landing were required shortly after takeoff. Landing field requirements can more frequently limit an airplane’s allowable landing weight than the approach climb limitations. Again, however, unless the runway is particularly short, this is seldom problematical as the average landing weight at the destination seldom approaches the maximum design landing weight due to fuel burn off. This concludes the Aircraft Performance page. You can now go on to the Flight Planning page or test your knowledge at the FAA Principles of Flight test. |